SOLAR-TERRESTRIAL CYCLES
Creative Functions of Cycles
Predictable Phase-Shift in Solar-Terrestrial Cycles
by Theodor Landscheidt (Reprinted from 1989 Proceedings)
Recent research has shown that cycles are at the core of creativity. They form antagonistic centers of polar tension, the competing realms of which generate fractal boundaries, sites of instability where new forms emerge. This knowledge, when applied to cycles and boundaries in the solar system, makes it possible to predict phases of instability, phase-shift, and emergence of new patterns in solar-terrestrial cycles.
Cycles are at the core of creativity. They form antagonistic centers of polar tension, sites of instability where new forms emerge.
he science of cycles deals not only with events that recur with reasonable regularity. More fundamental are the questions of where cycles come from and what are their cardinal functions. Cycles are often defined as a series of occurrences in which conditions at the end of a series are the same as they were at the beginning. Such mechanical identity, represented by sinusoidal waves, does not exist in Nature. Conditions at the end of cyclic processes are always different from those at the beginning and influence their further development. Part of the output of a completed oscillation acts as new input upon the next initial phase. Thus, feedback processes are a more appropriate model of cyclic functions than sinusoidal waves.
Intriguingly, recent research has shown that feedback cycles are an archetype of morphogenesis. It is established now that seemingly simple dynamic systems that comprise loops or cycles do not necessarily possess simple dynamic properties. Even extremely simple mathematical models of nonlinear dynamic feedback systems provide surprising evidence of unfathomable structural complexity. Take an initial number, the seed, square it, and add a constant. Feed the result, the output, back into this functional process as new input. Complete this cycle over and over again to see if some pattern emerges. The respective output values, when plotted in the plane of complex numbers, reveal the existence of so-called attractors that reign over separate domains into which they attract special output. Such attractors at zero and infinity, for instance, could represent the polar tension created by the antagonistic qualities of concentration and dissipation. Surprisingly, this discloses a hidden connection between feedback loops or similar cyclic features and centers of polar tension diametrically opposite in Nature.
FIG. 1. Boundary pattern, called Julia set, elaborated by H.O. Peitgen and P.H. Richter. Julia sets, a product of mathematical feedback cycles, carry incredibly complex dynamics emerging from boundaries between domains of antagonistic attractors that compete for influence. In border regions with polar tension, transition from one form of existence to another takes place: from order to disorder, magnetic to nonmagnetic state, or however the opposite entities are to be interpreted that meet at the boundary.
The proportions of the Mandelbrot set provide a wealth of clues to musical harmony, even the ratios of harmonics of cosmic cycles.
At the boundary between the realms of competing at-tractors, where their influence approaches zero as a limit, wonders of creativity emerge as patterns delicately poised between order and chaos. Computers can be used as a kind of microscope for viewing such boundaries. Fig. 1 shows an example of a microscopic boundary pattern made visible by H.O. Peitgen and P.H. Richter. Mathematically, it is a Julia set. Peitgen and Richter call it "seahorse tail." It winds down and down, going on forever. There is infinite regress of detail. The large tail encompasses complete smaller seahorses that show different features, thus representing a wealth of explicit forms.
At the core of those creative patterns that grow out of the boundary region exists a central form that represents the implicit order as we find it in cell nuclei. Zooming in for a close computer look discloses it. It is shown in Fig. 2 as elaborated by Peitgen and Richter. Mathematicians call it a Mandelbrot set after its discoverer. It regulates the form of different Julia sets, and thus may be looked upon as a pre-image of central regulation as performed in cell nuclei. The proportions of the Mandelbrot set provide a wealth of clues to musical harmony, including the major perfect chord. It has been shown that even the ratios of harmonics of cosmic cycles are precisely reflected in this core structure (Landscheidt 1989).
Those boundaries that separate the realms of antagonistic attractors which compete for influence are a model of reality. They represent the qualities of the phase boundary between magnetism and nonmagnetism, laminar flow and turbulent flow, cyclicity and noncyclicity, order and chaos, etc. Because of its fractal character, the border is infinitely long. By definition, it has infinitesimal width. Thus, its sensitivity is such that the stroke of a butterfly's wing can change the fate of macrocosmic systems, as American meteorologist E.N. Lorenz put it. This is why we do not know whether the solar system will continue to be stable or not. An infinitesimal difference in the initial conditions not accessible to calculation could lead to the dissolution of order in the planetary system. Physicist and mathematician Henri Poincar6 provided evidence of this as early as at the end of the 19th century. A wealth of boundaries with such qualities is to be found in the solar system. This refutes the often repeated argument that the energy level of any planetary influence on Sun or Earth is too low. It is inherent in the conditions of emergence of boundaries that their creative potential is irresolvably linked to instability. However, it is just this instability in dissipative systems far from the state of thermodynamic equilibrium that, according to Ilya Prigogine, Hermann Haken, and Erich Jantsch, can lead to a spontaneous formation of new structures out of germs of gestalt, or even out of chaos. If there is a boundary transition from one polar quality to the opposite one, instability will arise, but new patterns will emerge too. Our knowledge of the prototypical quality of boundaries can help us to judge the function of special boundary states and transitions properly. This applies to zero phases in cycles, critical days in biorhythms, the boundary between consciousness and subconsciousness, the transition from waking to sleeping with its Kekul6-ef-fects, creative acts of artists, eureka moments, mystic experience, birth, death, the balance in mathematical formulae, and interdisciplinary research. Mesocosmic man lives just on the boundary between the domains of the attractors microcosmos and macrocosmos. This might explain man's instability, the dominant quality of all borderlines between chaos and order, but also the unfathomable depths of man's creativity and destructiveness. A crisis in the development of individuals or collectives is again in boundary state. The Swiss writer Max Frisch put it thus: A crisis could be productive if only the tang of catastrophe would be taken from it.
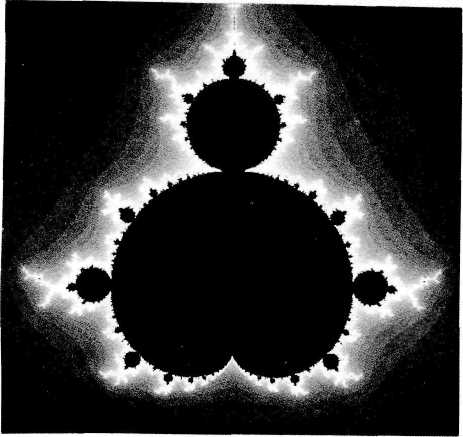
FIG. 2. Mandelbrot set, elaborated by H.O. Peitgen and P.H. Richter, which controls the structure of a wealth of diverse Julia sets. According to Peitgen and Richter, it reminds of the genetic organization in higher organisms. Its proportions express consonant intervals in musical harmony and the major perfect chord. Mandelbrot sets, as well as Julia sets, point to creative functions of feedback cycles.
The Earth's surface and the wealth of fractal structures growing out of this small boundary region is a further example. Such spherical surfaces are most interesting. Kepler thought them to be a perfect symbol of God's creative functions. Surfaces of material spheres are phase interfaces where symmetry breaking occurs; the invariance of vertical translation relative to the surface is no longer valid (Krueger 1984). New structures can emerge. The Sun's surface, too, shows such boundary qualities. It separates the realms of two competing attractors: one that represents contraction and transforms dying suns into neutron stars or even black holes, and another one that represents expansion and dissipating radiation. The borderline between these realms, the Sun's surface, shows the attributes of all boundaries: instability and a wealth of structures. The complex patterns of solar activity— sunspots, faculae, prominences, eruptions, and flares — arise from the Sun's thin, unstable surface layer. This surface activity is linked up with the Earth's surface activity. This is together a connection between two boundary regions in the defined sense.
It has been shown at international conferences of astronomers, geophysicists, and climatologists that impulses of the torque (IOT) in the Sun's irregular oscillations about the invisible center of mass of the solar system, induced by the giant planets, regulate the incidence of energetic solar eruptions and their terrestrial response (Landscheidt 1988a). Strong impulses of the torque occur when the Sun's center, the center of mass, and Jupiter, the weighty center of the world of planets, are in line. These heliocentric conjunctions of Jupiter and the center of mass again are a boundary phenomenon. They mark a zero phase in the Sun's motion about the center of mass, in which the torque acting on the Sun reaches zero, changes sign, and shows a more or less sharp increase in the new direction. The Sun changes from approaching the center of mass to receding from it, or vice versa. Just this boundary phenomenon releases instability together with a wealth of new patterns in the Sun's surface that is followed by corresponding effects in the Earth's surface region. These terrestrial responses range from geomagnetic storms to abundance in wildlife and economic change.
The Sun's irregular oscillations about the invisible center of mass of the solar system regulate the incidence of energetic solar eruptions and their terrestrial responses, which range from geomagnetic storms to abundance in wildlife and economic change.
Fig. 3 shows the cyclic variation in Canadian lynx abundance for the years 1735 to 1969. The data are based on records of the offerings of lynx skins by trappers, particularly to the Hudson's Bay Company. As the efforts of trappers to earn a livelihood are fairly constant, biologists feel that the records of skin offerings constitute a rather reliable index of the abundance of lynx. The ordinate axis in Fig. 3 indicates the yearly number N of offered lynx skins. Flat triangles mark the epochs of conjunctions of Jupiter with the center of mass. These epochs show an obvious connection with extrema in the lynx abundance. The pattern, however, is rather complex. There are longer periods with epochs that coincide with peaks, as before 1789; other protracted periods with epochs that concur with troughs, as after 1867; and phase jumps from minima to maxima, as before 1823 and 1867. Such phase changes appear in almost all time series of solar and terrestrial data, and are thought to pose intricate questions. The First three arrows in the plot point to periods of instability resulting in phase jumps that started about 1789,1823, and 1867. Even these periods of change show a distinct pattern: the epochs of Jupiter conjunctions on the left of the respective arrows always coincide with a maximum in lynx abundance, whereas the epoch on the right concurs with a minimum. This regular pattern of instabilities yields different results, depending on whether the foregoing epochs of Jupiter conjunctions go along with maxima or mim'ma in the time series. Only the instability event starting in 1933 resulted in a different pattern: there was no phase change, but an anomalous period with very small numbers of lynx and poor variations in the abundance.
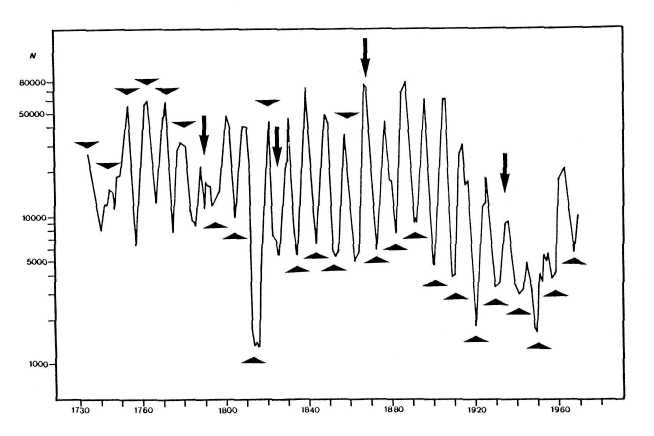
FIG. 3. Cyclic variation in Canadian lynx abundance, 1735 to 1969 (after E.R. Dewey). The ordinate axis indicates the yearly number N of offered lynx skins. Flat triangles mark the epochs of special constellations when the Sun's center, the center of mass of the solar system (CM), and the giant planet Jupiter are in line (Jupiter-CM conjunctions). These epochs are related to extrema in the lynx abundance. The phase pattern of this relationship, or major anomalies in the distribution, depend on major solar instability events, the starting epochs of which (1789, 1823,1867, and 1933) are designated by arrows. Major instability events occur when the center of mass, an attractor, stays for years in or near the Sun's surface, a boundary region.
Fortunately, our knowledge of the fundamental quality of boundaries, derived from mathematical models of feedback cycles and physical phase transitions, when combined with astronomical facts, enables us to explain and predict such instability events. The center of mass of the solar system is an attractor as defined above.
Hermann Haken, the founder of synergetics, a new discipline dealing with nonequilibrium phase transitions and self-organization in physics and biology, has focused attention on profound analogies between completely different systems when they pass through an instability and then build up new macroscopic spatio-temporal patterns. Haken explicitly states that the equations of celestial mechanics follow the rules of synergetics. He stresses that centers of mass of systems of astronomical bodies play an important part in nonequilibrium phase transitions, because they act as order parameters that function like at-tractors. As has been shown already, the Sun's surface is a boundary in terms of the morphology of nonlinear dynamic systems. Thus, it makes sense that the major instability events starting about 1789,1823, and 1867, and later about 1933 and 1968, just occurred when the center of mass kept staying in or near the Sun's surface for several years.
When the Sun approaches the center of mass (CM), or recedes from it, there is a phase when CM passes through the Sun's surface. Usually, this is a fast passage, as the line of motion is steeply inclined to the surface. There are rare instances, however, when the inclination is very weak, when CM runs nearly parallel with the Sun's surface, or oscillates about it, so that CM keeps staying near the surface for several years. Fixing the epochs of start and end of such periods involves some arbitrariness. The following definition is in accordance with observation and meets all requirements of practice: Major solar instability events occur when the CM keeps staying continually within the range 0.9-1.1 solar radii for 2.5 to 8.5 years, and additionally within the range 0.8-1.2 solar radii for 5.5 to 10 years. The giant planet Jupiter is again involved. In most cases, major instability events are released when Jupiter is stationary near CM. The first, sharper criterion yields the following periods:
1789.7-1793.1 (3.4yr) 1823.6-1828.4 (4.8 yr) 1867.6-1870.2 (2.6 yr)
1933.8--1937.3 (3.5 yr) 1968.4-1972.6 (4.2 yr) 2002.8--2011.0 (8.3 yr)
The first decimal is given only to relate the results rather exactly to the criterion. The epochs of the onset and the end of the phenomenon cannot be assessed with such precision. The second, weaker criterion yields periods which begin earlier:
1784.7-1794.0 (9.3 yr) 1823.0--1832.8 (9.8 yr) 1864.5-1870.9 (6.4 yr)
1932.5-1938.3 (5.8 yr) 1967.3--1973.3 (6.0 yr) 2002.2-2011.8 (9.6 yr)
Henceforth, the starting periods 1789, 1823, etc,.of the first criterion will be quoted.
In case of major instability events that affect the Sun's surface and the incidence of features of solar activity displayed in this thin, sensitive layer, the instability seems to spread out in the planetary system and seize all events in time series that are connected with the Sun's activity. The instability pattern in the abundance of lynx is only one example of this effect. The rhythm induced by Jupiter conjunctions with the center of mass, however, does not show any disturbances if there are no major instability events.
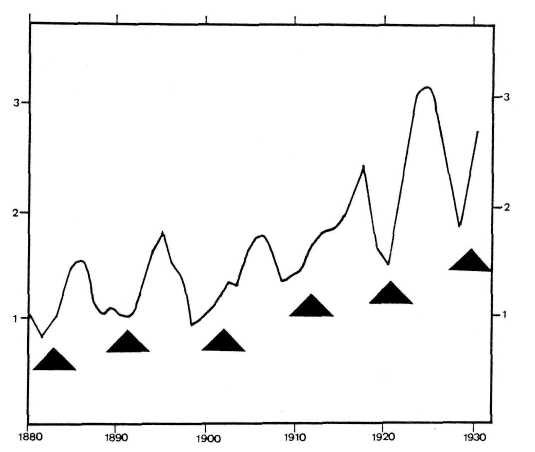
Fig. 4. Cycle of Atlantic salmon abundance (after E.R. Dewey). The ordinate axis indicates the catch of salmon per rod per day in the Canadian Restigouche Salmon Club, 1880 to 1930. Flat triangles mark epochs of Jupiter-CM conjunctions. Their consistent connection with minima in the abundance is in phase with that of the Canadian lynx. This relationship was not disturbed by phase anomalies, as there were no major solar system instability events during the period of observation.
Fig. 4 presents a cycle in Atlantic salmon abundance. The ordinate axis indicates the catch of salmon per rod per day in the Canadian Restigouche Salmon Club, 1880 to 1929. Flat triangles indicate the epochs of Jupiter conjunctions. The consistent connection with minima in the abundance is in phase with that of the Canadian lynx and the Chinch bug in Illinois. Calculations show that there was no major instability event between 1880 and 1929.
Major instability events seem to affect nearly everything in the solar system. They not only mark turning points in long-term solar activity, but also influence planets as a whole. Fig. 5 shows the response of the secular variation in the Earth's magnetic field. The upper curve presents the time rate of change of the northern component of the field. The triangles mark starting epochs of periods of major instability in the Sun's surface, about 1933 and 1968, that coincide with turning points in the curve. The geophysical literature is replete with papers on the geomagnetic jerk around 1968.
The years that followed 1789,1823, 1867,1933, and 1968 were periods of radical change and revolution, a break-down of old structures and the emergence of new forms and ideas. The next major instability event will start about 2002.
Human activity is involved too. Economy is an expression of such activity. As has been shown at the Foundation's 1988 Annual Conference, phase shifts emerge in various economic time series when major instability events are released. Maxima or minima in economic data that were in phase with the epochs of heliocentric conjunctions with the center of mass, turn out of phase when the center of mass remains in the Sun's surface for a longer period. As the conference proceedings demonstrate, this relationship is valid for American and European stock prices, U.S. pig-iron prices, and variations in gross national products (Landscheidt 1988ft, 1988c).
The special constellation of Sun and planets that makes the center of mass and the Sun's surface coalesce is, too, reflected in the fields of sociology, art, and science. The years that followed 1789, 1823, 1867,1933, and 1968 were periods of radical change and revolution, a breakdown of old structures and the emergence of new forms and ideas. The following examples give an impression of the turning-point function of these epochs, a proper expression of the basic quality of boundary effects as explained in the beginning:
About 1789 to 1793:
Great French revolution
spread of modern democracy
first modern constitution in the U.S.
invention of lighting gas Mozart, The Magic Flute
About 1823 to 1828:
Monroe doctrine
end of the Spanish empire in South America; Simon Bolivar
Greek war of independence against the Turkish rule
Decembrist conspiracy in Russia
non-Euclidian geometry
foundation of thermodynamic theory
Ohm's law in electricity
Brownian movement
first synthesis of an organic compound (urea) from inorganic substance
invention of steam locomotive, electromagnet, sewing machine, ship's propeller, aniline dye
Beethoven, Ninth Symphony, Missa Solemnis; Schubert, Seventh Symphony
About 1867 to 1870:
Alaska bought by U.S.
nihilism in Russia
Karl Marx, The Capital
foundation of first Social Democratic Party
Maxwell's equation describing the interrelation of electric and magnetic fields
concept of periodic table of chemical elements
Kekule's concept of six-carbon benzene ring
invention of dynamo, spark-ignition engine, typewriter,
rotary press printing, reinforced concrete, dynamite impressionism in art
About 1933 to 1937:
Great economic depression; collapse of the world market
Stalin's dictatorship; forced collectivisation of agriculture
beginning of Japan's Far Eastern expansion
breakthrough of Hitler's national socialism
Goedels revolution in logic
discovery of neutron, positron, meson, heavy hydrogen
creation of electron-positron pairs from energy
discovery of nucleic acid in cell nuclei
invention of television, jet engine, electron microscope
About 1968 to 1972:
Upheavals and rebellions of students all over the world spread of hippies
Cultural revolution in China six-day Arab-Israeli war
new economic structures in Czechoslovakia, suppressed by Russian invasion
turning point in Vietnam
space travel; astronauts on the Moon
Glomar Challenger expedition, plate tectonics
first AIDS infections
ecological movement
Gnostics of Princeton
Pop art
About 2002 to 2011:
The next major instability event will start about 2002 and last till 2011. This is an exceptionally long period. It is impossible to predict the details of its historic effects. But the basic quality of all boundary functions will be evident: the years past 2002 will prove to be another turning point, a period of instability, upheaval, agitation, and revolution, that ruins traditional structures, but favors the emergence of new patterns in society, economy, art, and science. Furthermore, the rhythm in terrestrial time series that show a connection with the Sun's activity will change again. Perhaps people in the beginning third millennium will be better prepared to realize the dangers and chances of cosmic periods of change like these. Possibly, the results of interdisciplinary research in the nature of cycles, presented here, will contribute to such awareness.
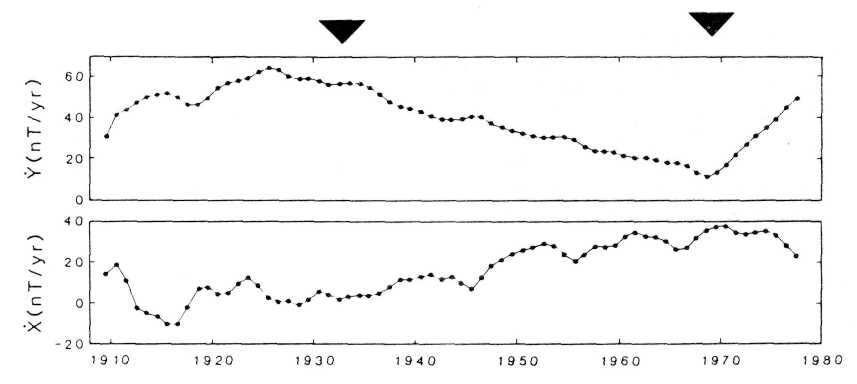
FIG. 5. Secular variation in the Earth's magnetic field (after M.G. McLeod). The top curve presents the time rate of change of the northern component of the Earth's magnetic field. Triangles mark the starting epochs, 1933 and 1968, of major instability events in the solar system. They seem to have left their mark in the secular variation of the geomagnetic field.
References Cited
Dewey, E.R., 1973. Cycles. Manor Books, New York.
Haken, H., 1978. Synergetics. Springer, Berlin, Heidelberg, New York.
Jantsch, E., 1980. The Self-Organizing Universe. New York.
Krueger, F.R., 1984. Physik und Evolution. Paul Parey, Berlin, Hamburg.
Landscheidt, T., 1988a. Solar rotation, impulses of the torque in the sun's motion, and climatic variation. Climatic Change 12:265-295.
Landscheidt, T., 1988ft. Conjunctions of Jupiter with the center of mass: a cosmic indicator of turning points in economic cycles. Proceedings, Foundation for the Study of Cycles Annual Conference, Irvine, California, p. 79-81.
Landscheidt, T., 1988c. Multidisciplinary forecast of stock prices. Proceedings, Foundation for the Study of Cycles Annual Conference, Irvine, California, p. 75-78.
Mandelbrot, B.B., 1975. Les objets fractals. Flammarion, Paris.
McLeod, M.G., 1985. On the geomagnetic jerk of 1969. /. Geophys. Res. 90:4597-4610.
Peitgen, H.O.; Richter, P.H., 1986. The Beauty of Fractals: Images of Complex Dynamical Systems. Springer, Berlin, Heidelberg, New York.
Poincare, H., 1889. Sur le probleme des trois corps et les equations de la dynamique.
Published on http://bourabai.narod.ru/ according permission of Frau Christiane Landscheidt
Вещество и поле не есть что-то отдельное от эфира, также как и человеческое тело не есть что-то отдельное от атомов и молекул его составляющих. Оно и есть эти атомы и молекулы, собранные в определенном порядке. Также и вещество не есть что-то отдельное от элементарных частиц, а оно состоит из них как базовой материи. Также и элементарные частицы состоят из частиц эфира как базовой материи нижнего уровня. Таким образом, всё, что есть во вселенной - это есть эфир. Эфира 100%. Из него состоят элементарные частицы, а из них всё остальное. Подробнее читайте в FAQ по эфирной физике.
|
|
 |
